---

Kraken Fish

A Kraken Fish is a fish pattern which is connected to a candidate elimination cell (CEC) in such a way that placing this candidate would reduce the secondary set to a size smaller than the defining set.

Here is a diagram depicting a Kraken X-Wing. It has 2 CECs.

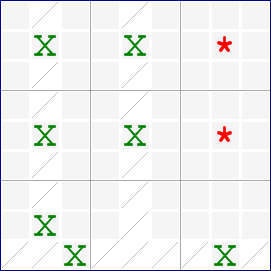
There is a potential X-Wing in columns 2 & 5 and rows 2 & 5. The candidate in r8c2 acts as a fin, but because it does not share a box with one of the candidate forming the X-Wing, it does not cause any eliminations that would normally occur in a Finned X-Wing. The pattern has 2 columns covering 3 rows.

Now consider what would happen if either r2c8 or r5c8 would contain digit X. Both would eliminate all candidate on their own row and through the strong link in row 9, the candidate in r8c2, leaving a pattern with 2 columns covering 1 row, an impossible situation.

A Kraken-related Extended 2-String Kite Example

Next consider the example below. This is identical to the Kraken X-wing example except the candidate has benn removed from cell r2c2. The resultant pattern is a 2-string kite consisting of conjugate pairs AB and CD and an additional conjugate pair EF. This is an example of a more general technique called the extended 2-string kite technique.

.-------.-------.-------.
| . - . | . - . | . . . |
| . - . | . F . | . * . |
| . - . | . - . | . . . |
:-------+-------+-------:
| . - . | . - . | . . . |
| . D . | . E . | . * . |
| . - . | . - . | . . . |
:-------+-------+-------:
| . - . | . - . | . . . |
| . C . | . - . | . . . |
| - - A | - - - | - B - |
'-------'-------'-------'

How it works. Since A and C are peers, at most only one of them can be X. This means that either or both of their conjugates B and D must be X. If B is X then r25c8 cannot be X. If B is not X, then D must be X and its peer E must be not X. Therefore E's conjugate F must be X. Therefore r25c8 cannot be X. So you get the same eliminations as the Kraken X-wing. A similar argument holds if r5c2 had been eliminated instead of r2c2. This means that either r2c2 or r5c2 but not both can be eliminated from the Kraken X-wing pattern without changing the results. The extended 2-string kite pattern occurs more often then the Kraken X=wing in puzzles.

Connection Types

In the example, the CECs are connected to the pattern with a direct weak link and an indirect connection via a strong link. However, there are several other methods to connect the CEC to the fish pattern.

• Strong links
• Grouped strong links
• Almost Locked Sets

As a result, the CEC does not even need to be a candidate for the same digit as the pattern. The following diagram shows how an X-Wing pattern for digit X can eliminate a candidate for digit Y.

.  -  .  | .  -  .  |  .  .  .
.  X  .  | .  X  .  |  .  XY .
.  -  .  | .  -  .  |  .  .  .
---------+----------+---------
.  -  .  | .  -  .  |  .  .  .
.  X  .  | .  X  .  |  .  .  .
.  -  .  | .  -  .  |  .  .  .
---------+----------+---------
.  -  .  | .  -  .  |  .  .  .
.  X  .  | .  -  .  |  .  .  .
.  -  XY | .  -  .  |  . CEC .

In this example, a similar Finned X-Wing is present, but r2c8 and r9c3 are both bivalue cells with candidates XY. Placing Y in the CEC at r9c8 would force both these cells to X and reduce the fish pattern to a single row and 2 columns.

External Link

• Kraken Fish (New Sudoku Players' Forums)

See Also

• Fish

This page was last modified 18:21, 12 July 2010.  

---